Constraint Solvers¶
Commutation Constraints¶
-
qecc.solve_commutation_constraints(commutation_constraints=[], anticommutation_constraints=[], search_in_gens=None, search_in_set=None)[source]¶ Given commutation constraints on a Pauli operator, yields an iterator onto all solutions of those constraints.
Parameters: - commutation_constraints – A list of operators
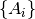 such
that each solution
such
that each solution  yielded by this function must satisfy
yielded by this function must satisfy
![[A_i, P] = 0](_images/math/faa5625b24f2e1148704ec6c1f9cef29e4a94ce8.png) for all
for all 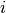 .
. - anticommutation_constraints – A list of operators
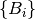 such
that each solution
such
that each solution  yielded by this function must satisfy
yielded by this function must satisfy
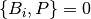 for all
for all 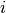 .
. - search_in_gens – A list of operators
 that generate
the group in which to search for solutions. If
that generate
the group in which to search for solutions. If None, defaults to the elementary generators of the pc.Pauli group on qubits, where
qubits, where
 is given by the length of the commutation and anticommutation
constraints.
is given by the length of the commutation and anticommutation
constraints. - search_in_set – An iterable of operators to which the search for
satisfying assignments is restricted. This differs from
search_in_gensin that it specifies the entire set, not a generating set. When this parameter is specified, a brute-force search is executed. Use only when the search set is small, and cannot be expressed using its generating set.
Returns: An iterator
itsuch thatlist(it)contains all operators within the group given by
given by search_in_gens, consistent with the commutation and anticommutation constraints.This function is based on finding the generators of the centralizer groups of each commutation constraint, and is thus faster than a predicate-based search over the entire group of interest. The resulting iterator can be used in conjunction with other filters, however.
>>> import qecc as q >>> list(q.solve_commutation_constraints(q.PauliList('XXI', 'IZZ', 'IYI'), q.PauliList('YIY'))) [i^0 XII, i^0 IIZ, i^0 YYX, i^0 ZYY] >>> from itertools import ifilter >>> list(ifilter(lambda P: P.wt <= 2, q.solve_commutation_constraints(q.PauliList('XXI', 'IZZ', 'IYI'), q.PauliList('YIY')))) [i^0 XII, i^0 IIZ]
- commutation_constraints – A list of operators